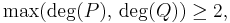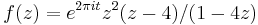Classification of Fatou components

In mathematics, if 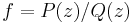$f = P(z)/Q(z)$ is a rational function defined in the extended complex plane, and if

$\max(\deg(P),\, \deg(Q))\geq 2,$

then for a periodic component $U$ of the Fatou set, exactly one of the following holds:

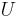$U$ contains an attracting periodic point
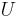$U$ is parabolic
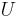$U$ is a Siegel disc
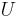$U$ is a Herman ring.

One can prove that case 3 only occurs when f(z) is analytically conjugate to a Euclidean rotation of the unit disc onto itself, and case 4 only occurs when f(z) is analytically conjugate to a Euclidean rotation of some annulus onto itself.

1 Examples
- 1.1 Attracting periodic point
- 1.2 Herman ring
2 References
3 See also

Examples

Attracting periodic point

The components of the map 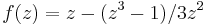$f(z) = z - (z^3-1)/3z^2$ that contains the attracting points that are the solutions to 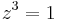$z^3=1$ . This is because the map is the one to use for finding solutions to the equation 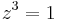$z^3=1$ by Newton-Raphson formula. The solutions must naturally be attracting fixed points.

Herman ring

The map

$f(z) = e^{2 \pi i t} z^2(z - 4)/(1 - 4z)\$

and t = 0.6151732... will produce a Herman ring.^[1] It is shown by Shishikura that the degree of such map must be at least 3, as in this example.

References

Lennart Carleson and Theodore W. Gamelin, Complex Dynamics, Springer 1993.
Alan F. Beardon Iteration of Rational Functions, Springer 1991.

^ Milnor, John W. (1990), Dynamics in one complex variable, arXiv:math/9201272

Classification of Fatou components

Contents

Examples

Attracting periodic point

Herman ring

References

See also